5-6 Double and Half Argument Properties
Hooray! I'm so excited that it's my turn to blog once again!
Now that we have learned all about all the other properties in the chapter, it is time to learn some more. This section focuses on the double and half argument properties.
You use the double argument properties when you are writing cos2x or cos(x+x), and the other trigonometric functions. Similarly, from these double argument properties, you can derive the half argument properties.
We use these properties for many different purposes, including finding the exact values without the use of our calculators....
Here are the properties in this section:
The Products and Squares of Cosine and Sine

We use thes when you have a sine and a cosine, or two cosines and two sines.
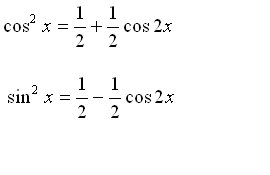
Example of when you use the products and squares of cosine and sine:

The Double Argument Properties (the important part)
note: we have to memorize the sine and cosine equations
Double Argument Property for Sine
sin 2A = 2sinAcosA
Double Argument Properties for Cosine
(yes, there are multiple equations for cosine)
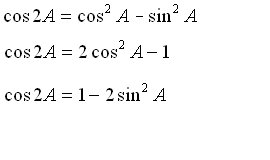
Double Argument Properties for Tangent
(although we don't have to know it)
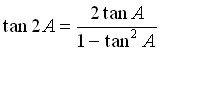
Example of when you use the double argument properties:

The Half Argument Properties for Sine, Cosine, and Tangent

Example of how to use the half argument property:
Find the exact value of sin 15° → think of it as half of 30
choose the positive because the angle is in the first quadrant, where sin is positive
So...basically, that's the lesson!
Here is a link just incase you want some extra help.... http://www.math.ucdavis.edu/~marx/doubang2Pr/doubang2Pr.html
My Favorite Part...the personalization!
Always remember to check the flavors of the day!: http://www.21choices.com/pasadena.html
and also....
the best place EVER for cupcakes is Sprinkles of course!
http://www.sprinklescupcakes.com/
a picture to show you the goodness...
the yummy holiday cupcakes!
Paul...you are next!!!
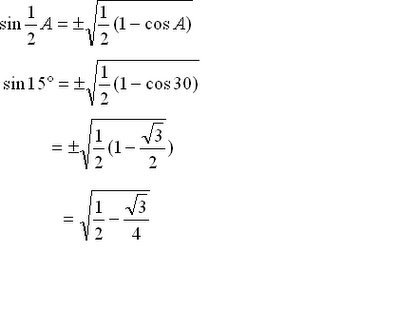

1 Comments:
Christina, this was really helpful. Personally, I think this section was the hardest one we've had so far, and you did an awesome job of explaining it.
Post a Comment
<< Home